![- n
G = {V ⊂ C ((T)) | dim (V ∩ T C[[T ]]) = n,∀n ≫ 0 }](work_done18x.png)
An algebraic construction for the KP Hierarchy can be given analogous to the “exp” map for Drinf’eld modules. The infinite Grassmannian is described as follows:
![- n
G = {V ⊂ C ((T)) | dim (V ∩ T C[[T ]]) = n,∀n ≫ 0 }](work_done18x.png)
Let X be any variety and p a smooth point on it. This gives us a base point n ⋅ p in Symn(X), the n-fold symmetric product of X with itself. We then have natural base point preserving maps Symn(X) → Symn+k(X) obtained by adding the zero-cycle k ⋅ p. The direct limit

has a natural multiplication induced by the morphisms
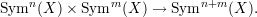
If X is 1-dimensional this group G acts on 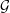 and the flow associated with this
action is then the KP flow.
and the flow associated with this
action is then the KP flow.