


Next: 6.1 Algebraic Numbers
Up: Some Lectures on Number
Previous: 5.3 Primality Certificates
We can look at the factorisation problem as the study of the group of
non-zero rationals; writing every element in terms of the generators
(the prime numbers and -1) and taking into account the relation
(- 1)2 = 1. The study of the unit group in
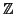 /N
/N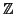 can be
identified with the study of a suitable quotient of a suitable
subgroup (elements prime to N) of this group. We now ask how
this group can be generalised. One natural idea is to use algebraic
number fields. An algebraic number is an ``object'' (we will be more
specific later) that satisfies a polynomial equation with rational
(equivalently integer) coefficients (we should actually insist on
irreducibility of the equation). We can represent such objects as we
will see below. However, it turns out that studying groups of
algebraic numbers is not quite the same as studying the generalised
factorisation problem; that involves the study of divisors or
ideals and their groups.
can be
identified with the study of a suitable quotient of a suitable
subgroup (elements prime to N) of this group. We now ask how
this group can be generalised. One natural idea is to use algebraic
number fields. An algebraic number is an ``object'' (we will be more
specific later) that satisfies a polynomial equation with rational
(equivalently integer) coefficients (we should actually insist on
irreducibility of the equation). We can represent such objects as we
will see below. However, it turns out that studying groups of
algebraic numbers is not quite the same as studying the generalised
factorisation problem; that involves the study of divisors or
ideals and their groups.
Subsections
Kapil Hari Paranjape
2002-10-20