


Next: New Functions from old
Up: Functions, continuity and differentiability
Previous: Definitions
We show the important properties called the intermediate value
property and extremal value property of continuous functions. We also
deduce the clutch of theorems called mean value theorem, Rolle's
theorem and so on for differentiable functions.
The following important property of continuous functions will be used
all the time.
Exercise 39
Let f (x) be a continuous function and {xn} be a sequence
converging to c, then {f (xn} is a sequence converging to
f (c). (Hint: Examine the condition for continuity near c).
Let f (x) be continuous for x satisfying
a 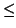 x
x 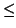 b. Let c
be a real number lying between f (a) and f (b) we want to show that
c is a value of f; in other words any number intermediate to two
values is itself a value.
b. Let c
be a real number lying between f (a) and f (b) we want to show that
c is a value of f; in other words any number intermediate to two
values is itself a value.
Exercise 40
Let
s be the least upper bound of the set
Show that
f (
s) =
c.(Hint: To show that
f (
s)
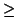 c
c take a sequence
of points approaching
s from above).
Now let C be the least upper bound of the values of f (x), i. e. it is the least upper bound of the set
{f (x)| a 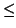 x
x 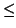 b}. The
C is an extremal value for f.
b}. The
C is an extremal value for f.
Exercise 41
Show that
C =
f (
x) for some
x in the range
a 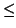 x
x 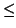 b
b. (Hint: We have a sequence {
xn} so that
f (
xn) converges
to
C; by the section on sequences this sequence has a convergent
subsequence).
The following property of differentiable functions is very important
Exercise 42
Let
f (
x) be differentiable for
x satisfying
a 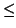 x
x 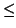 b
b. Let
s be such that
f (
s) is an extremal value for
f. Then
f'(
s) = 0. (Hint: Examine the condition for differentiability near
s).
Now suppose that f (x) is differentiable in the range a < x < b and
continuous at the endpoints a and b as well. Suppose that
f (a) = f (b) = 0. There is a point s where f attains its maximal
value; similarly there is a point t where f attains its minimal
value. If s is the point a or b then
f (x) 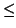 0 and if t is
the point a or b then
f (x)
0 and if t is
the point a or b then
f (x) 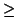 0. Thus in case both of these
occur then f (x) = 0 for all x; then let c = (a + b)/2. Otherwise let
c be any one of s and t which is not a or b. Thus we have a
point where f'(c) = 0.
0. Thus in case both of these
occur then f (x) = 0 for all x; then let c = (a + b)/2. Otherwise let
c be any one of s and t which is not a or b. Thus we have a
point where f'(c) = 0.
Exercise 43
For a general function
g(
x) which is differentiable in the range
a <
x <
b and continuous at the endpoints we apply this to the
function
f (
x) =
g(
x) +
 .
. (
x -
a)
to show that there is a point
c where
g'(
c) =




Next: New Functions from old
Up: Functions, continuity and differentiability
Previous: Definitions
Kapil H. Paranjape
2001-01-20
 x
x  b andf (x)
b andf (x)  c}
c}
 . (x - a)
. (x - a)
