I was once travelling by train when my co-passenger asked me (as co-passengers in India tend to do!) what my profession was. When I said “Research in Mathematics” he probed further and extracted the fact that I do research in “Geometry”. To this he responded,
“Surely, there is nothing more left to do in Geometry!”
This misconception has something to do with the way we teach mathematics. Each thing we teach seems to be complete in itself. The geometry that we learnt in high-school seemed to be quite complete; especially since the sticky bit about the Parallel Postulate is carefully hidden away!
In the following paragraphs, I will present a quick summary1 of geometry as it developed beyond Euclid up until Cartan’s (‘gauge’) theory of connections and principal bundles. The latter seemed to be a complete theoretical framework within which geometry had to be studied, until Mikhail Gromov entered the scene. The discussion of some of Gromov’s ideas will be the topic of the next section.
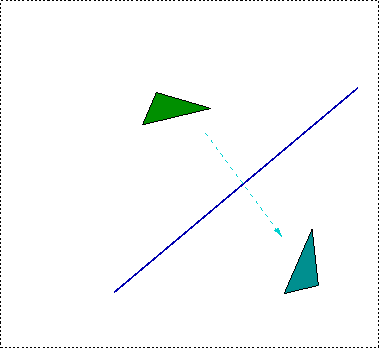
The figures that we draw in high-school geometry classes have properties that are unchanged under the operation of reflection in a line.

In fact, each construction in Euclidean geometry is determined by some finite collection F of points. The geometric figures determined by F and F′ are congruent if F can be reached from F′ through a series of reflections (in lines).

It was perhaps Sophus Lie (see inset2 ) who first realised that the converse is also true. He concluded that symmetry lies at the the basis of high-school geometry and used the mathematical formulation of symmetry in terms of the theory of groups to restate the axioms of high-school geometry.
A Group is a set G, with a special element e called the identity element, a binary operation (⋅) called group multiplication and a unary operation ()-1 called inversion such that:
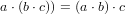

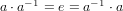
Lie realised that each sequence of reflections gives a transformation of the plane; the figure shows how rotations and translations can be made out of reflections.
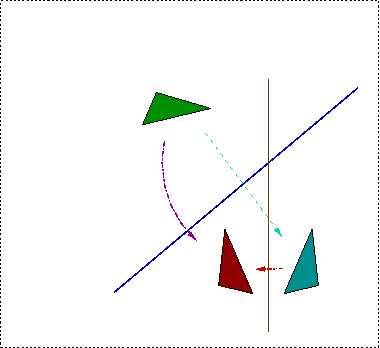
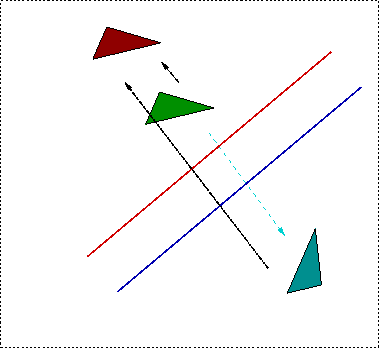
The collection of such transformations forms a group which is called the group of (plane) Euclidean transformations; actually, every element of this group is a product of at most three reflections. The usual properties of geometric figures are precisely those that are invariant under the action of this group. These properties include distances, angles, areas, and so on.

Felix Klein (see inset) realised that a similar description was applicable to the non-Euclidean geometry of Bolyai and Lobachevsky. So he turned the problem on its head and thought of a geometry as a space with the property that there is a group of transformations of the space which takes any given point to any other given point; this was his way of formulating the apparent homogeneity of (Platonic) physical space. The study of geometry is then the study of those properties of figures that are left invariant under this group of transformations.
The group of translations and rotations can intuitively be seen as ‘continuous’ groups. Lie had already undertaken the enormous task of studying continuous groups and their invariants.
A quite different kind of group is the group of Frieze patterns and wallpapers that has been used by artists and architects (especially those from India and West Asia) for centuries.
 |  |  |  |
 |  |  |  |
Klein realised that the systematic study of the corresponding ‘discontinuous‘ groups (or ‘discrete’ groups) would also play an important role in geometry. As a result he took up the task of studying groups which created ‘tilings’ of the homogeneous geometries that Lie and he had introduced earlier. This combination of the study of ‘continuous’ and ‘discrete’ groups of transformations as the basis of geometry was later called Klein’s Erlangen Programme.

Some of the most beautiful mathematical drawings are to be found in the work of M. C. Escher (see inset for a self-portrait). His drawings visualised mathematical ideas and his work contains a number of fantastic and un-worldly pictures. One of the things that inspired him was the non-Euclidean geometry of Bolyai and Lobachevsky. We see below an example of one his beautiful designs based on non-Euclidean tilings.


Bernhard Riemann (see inset) had taken a rather different approach to geometry. His principal idea was that once we can measure speed, we can measure the distance travelled. Once we can measure distance travelled one can study ‘geodesics’ or shortest paths. (Actually Riemann had already learnt from his mentor Carl Friedrich Gauss that ‘geodesics’ are better thought of as ‘least energy paths’. This is something which drivers in Chennai would do well to learn!) The measurement of speed (now known as the Riemannian metric) is the fundamental geometric notion in what is now called Riemannian Geometry; all other geometric properties can be derived once the metric is known.
Just as the ‘real’ world does not exhibit ‘true’ mathematical homogeneity, Riemann’s geometries which were inspired by his view of the physical world had no obvious homogeneity. However, the ‘universal’ nature of physical laws does indicate that some kind of homogeneity must be present. It turns out that physical laws are best expressed as ‘infinitesimal’ homogeneity; i. e. the infinitesimal regions around any two points in space are identical. This is beautifully explained in Feynman’s essays on “The Character of the Physical Law”.

Elie Cartan (see inset) realised that ‘infinitesimal’ homogeneity can be realised as a suitable group of infinitesimal transformations. The ‘development‘ of this infinitesimal geometry into a space is given by Cartan’s structural equations. Following work of Hermann Weyl one can characterise the group by specifying a (finite) collection of tensors (or more generally spinors) which are invariant under this group of transformations. This led Weyl to define geometry in terms of ‘gauge theory‘ (which was later formulated by Ehresmann as the study of connections on a principal bundle).
The approach to geometry as the study of connections on a principal bundle on a manifold is well explained in the excellent book by Kobayashi and Nomizu. Again, like Euclidean geometry, it appears in this book to be a complete theory. It did indeed seem as if everyone was working in this formulation of (differential) geometry during the 1960’s and 70’s.

Mikhail Gromov found a way to study geometry beyond its formulation by Cartan and Weyl. To understand his ideas we begin with a description of non-Euclidean geometry in terms of Euclidean geometry.
The non-Euclidean plane defined by Bolyai and Lobachevsky can be identified with a half-plane H in Euclidean geometry which is bounded by a (Euclidean) line L; points on this line are not part of the geometry. The role of ‘straight-lines’ in this geometry is played by certain curves which (to avoid confusing terminology) we will refer to as the geodesics of the geometry. These are:
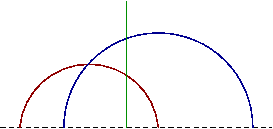
The usual axioms of Euclidean geometry, except the parallel postulate, are satisfied by this system of geodesics.
Now, if A, B and C are three points in the half-plane H, the consider the triangle ΔABC with vertex A; see the attached figure to understand the following argument. We can grow this triangle by moving B and C along AB and AC respectively. Even when we do this, the distance from the vertex A to the base BC (which is the height of the triangle) does not keep on growing (i.e. it remains bounded). Note that as the points B and C approach L their (non-Euclidean) distance from A (and thus the non-Euclidean size of the triangle) becomes infinite!
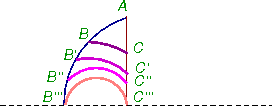
This is an important notion which Gromov called the ‘thin-ness’ of triangles.
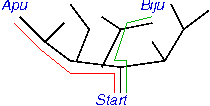
Consider the trails followed by ants on trees. Since ants are so efficient we can think of these as geodesics. Say Apu and Biju are two ants walking on a tree together. At some point the break-up and go on different branches, then the only way they can meet again is to come back to the branch point. This leads to really thin triangles since the distance from the vertex of the triangle to the base is fixed, without taking into account how far Apu and Biju go on their individual branches.
Comparing this example with the one before we see that the non-Euclidean plane shares some properties with trees.
The simplest way to tile the plane is to use square (or rectangular tiles). We can describe a walk (along the cracks between the tiles) as follows:
One can easily see that two people who start at the same place and make arbitrary choices at each crossing, still have good chance of meeting each other.

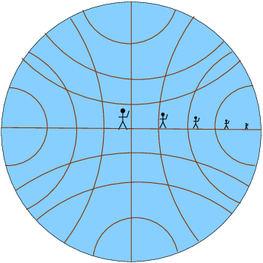
The result of following such a procedure on the non-Euclidean plane will result in something very different. The chance that the two people will meet each other is as small as the chance that both of them will end up re-tracing their steps!
A different way of saying this is that there is no rectangular tiling of the non-Euclidean plane. In fact, the discrete groups that are associated with tilings of the Euclidean plane are very different from those that occur for the non-Euclidean plane.
Consider the collection of all “words” in the symbols n, e, w, s. We can interpret each letter as an instruction to travel 1 metre in that direction. Each word gives us an algebraic formulation of the ‘lattice traversal’ described above. There is an obvious relation:
when the pairs ns, sn, ew or we occur in a word these can be dropped
In the Euclidean plane we see that we have additional relations like es = se, nw = wn and so on. However, in the non-Euclidean plane we get no additional relations.
In terms of group theory, the free group on two generators is the collection of all words:
If we add the relation for the Euclidean case, we get the free commutative (or Abelian) group.
We thus see that the discrete groups (or tilings) that occur in the the Euclidean case and non-Euclidean case are quite different.
The term hyperbolicity has become a common mathematical term used to describe the behaviour of geodesics in the non-Euclidean plane and other Riemannian spaces which exhibit similar behaviour. The key idea of Gromov was to give a way to identify the Large scale structure of a space in a way that hyperbolic spaces can be distinguished.
Prior to Gromov’s work in geometry, it was generally believed that continuous operations were the only way to observe qualitative properties of the space. For example, the study of topology has often been described as “rubber-sheet” geometry; such a sheet can be stretched but not torn.
Gromov realised that the large-scale structure of a space is preserved if some limited amount of “tearing” is allowed. He formulated the notion of quasi-isometry between spaces for this purpose. Consider two spaces X and Y where distances between pairs of points (in the same space) are denoted using d(,).
A (set-theoretic) map f : X → Y is said to be a (k,c) quasi-isometry if for any pair of points u, v in X we have

Here k and c are some positive (and possibly large!) constants.
As an aside, the prefixes quasi-, pseudo- and almost- generally make one feel queasy. We feel like asking why we should need to deal with these notions and not the real thing? One answer is that often mathematical objects are not congruent but only qualitatively similar. Having weaker notions than congruence allows us to see some common properties.
It turns out that quasi-isometry preserves the property of thin-ness of triangles. Moreover, the tree, non-Euclidean space and the free group (with an appropriate notion of distance) are all quasi-isometric!
Another nice aspect of this approach is that we need not restrict our study to spaces that are homogeneous. Even if there are quasi-isometries which can take one point of the space to another, one can think of the space as quasi-homogeneous and study groups of quasi-isometric transformations.
We need not restrict the scope of our study (as Cartan did) to geometries that are ‘infinitesimally’ homogeneous; in fact, the spaces do not need to have an infinitesimal structure at all! As long as there is a notion of distance we can apply Gromov’s methods to study it. This possibly explains why Gromov has become interested in applying his techniques to Biological systems; the latter have too many parameters to be analysed using Cartan’s geometry.
Returning to mathematics, Gromov’s method allows us to see a new connection between groups and the spaces on which they act and leads to ‘rigidity’ theorems like the one above which distinguishes between the groups acting on hyperbolic and Euclidean spaces.
This new confluence of Geometry and Group theory through the work of Gromov is the 3G technology of mathematics!