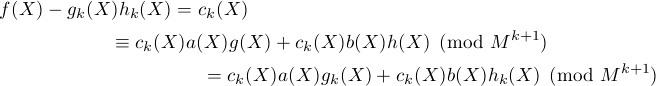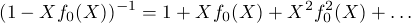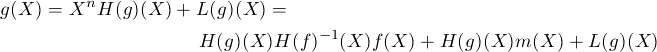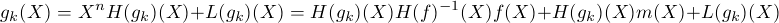FINITE RINGS
KAPIL HARI PARANJAPE
1. Finite (Dimensional) Rings
Let us first examine a ring A which is either finite or a finite dimensional algebra over a
field. It is clear that such a ring satisfies the descending chain condition for ideals. That
is to say any descending chain of ideals I0  I1
I1 
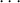 is constant after some i. A ring
satisfying this condition is also called an Artinian ring in honour of Emil Artin. The ring
also satisfies the ascending chain condition; any ascending chain of ideals is constant
after a finite stage. A ring satisfying this condition is called a Noetherian ring in honour
of Emmy Noether.
is constant after some i. A ring
satisfying this condition is also called an Artinian ring in honour of Emil Artin. The ring
also satisfies the ascending chain condition; any ascending chain of ideals is constant
after a finite stage. A ring satisfying this condition is called a Noetherian ring in honour
of Emmy Noether.
However, in this section we will only use the Artinian property to prove a structure
theorem. The Noetherian property will be a consequence! Thus the results will apply to
the larger class of all Artinian rings not just finite rings. The proofs in the latter case
could be marginally simplified.
1.1. Elements of an Artinian ring. Let x be an element of an Artinian ring A and
consider the sequence of ideals Ax  Ax2
Ax2 
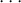 . By the descending chain condition this
sequence of ideals must become constant after some stage. In other words, there must be
an element y in A such that yxk+1 = xk for some (sufficiently large) integer
k.
Given such a y, let k be the smallest non-negative integer for which this equation
holds (with the convention that x0 = 1!). It is clear that if yx- 1 = 0 then x is a unit; in
other words x is an element of A×, the group of units of A. On the other hand if
yx - 1
. By the descending chain condition this
sequence of ideals must become constant after some stage. In other words, there must be
an element y in A such that yxk+1 = xk for some (sufficiently large) integer
k.
Given such a y, let k be the smallest non-negative integer for which this equation
holds (with the convention that x0 = 1!). It is clear that if yx- 1 = 0 then x is a unit; in
other words x is an element of A×, the group of units of A. On the other hand if
yx - 1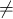 0 then we see that k > 0 and (yx - 1)xk-1
0 then we see that k > 0 and (yx - 1)xk-1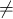 0; since (yx - 1)xk-1 . x = 0,
we see that x is a zero divisor. Thus every element of A is either a unit or a
zero-divisor.
0; since (yx - 1)xk-1 . x = 0,
we see that x is a zero divisor. Thus every element of A is either a unit or a
zero-divisor.
1.2. The Jacobson Radical of an Artinian ring. If x lies in an ideal I and
(yx - 1) lies in an ideal J, then I + J = A. On the other hand, the equation
(yx - 1) . xk = 0 shows that if (yx - 1) does not lie in a prime ideal P then x
must lie in P. An element of a ring that does not lie in any maximal ideal
is a unit (by applying Zorn’s lemma; however, this is not required for finite
rings or finite dimensional algebras). Thus (yx - 1) is a unit if and only if x
lies in all maximal ideals; the above equation shows that x is nilpotent in this
case.
Let J be the intersection all maximal ideals of a ring A. This is called the
Jacobson radical of the ring in honour of Nathan Jacobson. What we have shown
above is that the Jacobson radical of an Artinian ring consists of nilpotent
elements.
1.3. Prime ideals in an Artinian ring. Now, if P is a prime ideal of A to which x
does not belong, then the equation yxk+1 = xk says that (yx- 1) belongs to P so that x
becomes a unit modulo P. We can argue similarly for every element that does not belong
to P. Thus every prime ideal of an Artinian ring is maximal. We can also see this by
noting that the ideal consisting of all nilpotent elements of a ring is the intersection of all
its prime ideals.
1.4. Some basic facts about co-prime ideals. Let I and J be ideals in a ring A. We
say that they are co-prime if I + J = A. Let us state some basic facts about
co-primality:
- The product of co-prime ideals equals their intersection, i. e. I
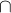 J = I .J.
J = I .J.
- Powers of co-prime ideals are co-prime, i. e. Ik + Jl = A for any positive
integers k and l.
- If I is co-prime to each of the ideals Ji, i = 1,...,n, then I is also co-prime
to the product
 iJi.
iJi.
All the above facts can be proved easily by observing that co-primality of I and J is
equivalent to the existence of an element x  I (respectively y
I (respectively y  J) such that x acts as
identity modulo J (resp. y acts as identity module I).
J) such that x acts as
identity modulo J (resp. y acts as identity module I).
For our purpose we note that this implies that for any collection M1, ..., Mk of
distinct maximal ideals we have
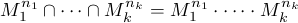
Moreover, if M is a maximal ideal distinct from these maximal ideals then
Mn 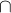 M1n1 .
M1n1 . . Mknk is a proper sub-ideal of M1n1 .
. Mknk is a proper sub-ideal of M1n1 . . Mknk. By the descending
chain condition applied in the case where the ni = 1 we see that there are only finitely
many maximal ideals in an Artinian ring.
. Mknk. By the descending
chain condition applied in the case where the ni = 1 we see that there are only finitely
many maximal ideals in an Artinian ring.
1.5. Annihilators and the ratio ideal. For an ideal I in a ring A we use AnnA(I) or,
where there is no ambiguity about the ring, Ann(I) to denote the annihilator of I; this
consists of the elements x in A such that x.I = 0. This is a particular case of the “ratio”
of ideals; if I 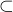 J are ideals of A we define (I : J) as the ideal consisting of elements
x in A such that xJ
J are ideals of A we define (I : J) as the ideal consisting of elements
x in A such that xJ 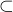 I; note that Ann(I) = (0 : I). The inclusion I
I; note that Ann(I) = (0 : I). The inclusion I 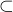 J is
strict if and only if (I : J) is strictly contained in A. Moreover, if J1
J is
strict if and only if (I : J) is strictly contained in A. Moreover, if J1 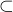 J2 then
(I : J2)
J2 then
(I : J2) 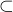 (I : J1).
(I : J1).
1.6. Minimal elements. Let x0 be a non-zero element of an Artinian ring A.
If I = Ann(Ax0) is not a maximal ideal, then there is a proper sub-ideal of
A such that J properly contains I; thus Jx0 is a non-zero proper sub-ideal
of Ax0. Let x1 be a non-zero element of Jx0. Then Ax1 is a non-zero proper
sub-ideal of Ax0. Continuing this process, we see that, the descending chain
condition for A implies that there must exist some multiple y of x0 with the
property that Ann(Ay) is a maximal ideal. We call such an element a minimal
element.
More generally, if I is any proper sub-ideal of A, then A/I is also a non-zero Artinian
ring. If x is an element of A whose image is a minimal element of A, then (I : Ax + I) is
a maximal ideal.
1.7. Nakayama-type lemma for Artinian rings. Let J again denote the Jacobson
radical of the ring A. As seen above Ann(J . I)  Ann(I); for an Artinian ring we will
show that that this is a strict inclusion whenever I is not the zero-ideal. It
will follow that J . I is strictly contained in I whenever I is not the zero ideal.
A result of this type for Noetherian (semi-)local rings is called Nakayama’s
lemma.
Since I is not the zero ideal, Ann(I) is a proper sub-ideal of the ring A. As seen above
there is an element x of A such that (Ann(I) : Ax + Ann(I)) is a maximal ideal M. It
follows that x . M . I = 0 so that x lies in Ann(M . I) but not in Ann(I). Since
J . I = K . M . I where K is the product of the remaining maximal ideals of A, we see
that the result follows.
Ann(I); for an Artinian ring we will
show that that this is a strict inclusion whenever I is not the zero-ideal. It
will follow that J . I is strictly contained in I whenever I is not the zero ideal.
A result of this type for Noetherian (semi-)local rings is called Nakayama’s
lemma.
Since I is not the zero ideal, Ann(I) is a proper sub-ideal of the ring A. As seen above
there is an element x of A such that (Ann(I) : Ax + Ann(I)) is a maximal ideal M. It
follows that x . M . I = 0 so that x lies in Ann(M . I) but not in Ann(I). Since
J . I = K . M . I where K is the product of the remaining maximal ideals of A, we see
that the result follows.
1.8. The nilpotency of the Jacobson radical. As above let J denote the Jacobson
radical of an Artinian ring A. By the result just proved J .Jr is a proper sub-ideal of Jr
if Jr is a non-zero ideal. The descending chain condition for ideals thus implies that Jr
must be the zero ideal for some r.
By the co-primality of the maximal ideals of A we see that
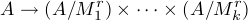
is an isomorphism when the product on the right runs over all maximal ideals of
A.
1.9. Artinian rings are Noetherian rings. If A is a ring and M is a maximal ideal,
then W = Mk/Mk+1 is a vector space over the field A/M. Any strictly decreasing
sequence of subspaces V i  V i+1 in W is of the form V i = Ii/Mk+1, where Ii
V i+1 in W is of the form V i = Ii/Mk+1, where Ii  Ii+1 is
a decreasing sequence of ideals. Thus, if A is an Artinian ring, this sequence must be
constant after a finite stage. It follows that W is a finite dimensional vector
space; we then conclude easily that any ideal of A/Mk is finitely generated.
Since A is itself a product of such rings, we see that any ideal in A is finitely
generated.
A ring where every ideal is finitely generated satisfies the ascending chain condition
for ideals. That is to say given an ascending chain of ideals I0
Ii+1 is
a decreasing sequence of ideals. Thus, if A is an Artinian ring, this sequence must be
constant after a finite stage. It follows that W is a finite dimensional vector
space; we then conclude easily that any ideal of A/Mk is finitely generated.
Since A is itself a product of such rings, we see that any ideal in A is finitely
generated.
A ring where every ideal is finitely generated satisfies the ascending chain condition
for ideals. That is to say given an ascending chain of ideals I0 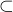 I1
I1 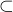
 , this sequence
is constant after some i. A ring satisfying this condition is also called an Noetherian ring
in honour of Emmy Noether. As we shall see later such rings arise naturally in algebraic
geometry.
, this sequence
is constant after some i. A ring satisfying this condition is also called an Noetherian ring
in honour of Emmy Noether. As we shall see later such rings arise naturally in algebraic
geometry.
1.10. The Nil radical of a Ring. If x is a non-nilpotent element of a ring R, then Rx
is a non-zero ring. By an application of Zorn’s lemma (which is not required if the ring R
(and hence Rx) is Noetherian), there is a maximal proper ideal M of Rx. The kernel of
the natural homomorphism R 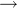 Rx/M is a prime ideal since the latter ring is a field.
We have thus shown that any non-nilpotent element is in the complement of
some prime ideal; the converse, that a nilpotent element belongs to all prime
ideals, is also clear. Hence the intersection of all prime ideals is also the ideal
consisting of all nilpotent elements of a ring; this ideal is called the nil radical of the
ring.
Rx/M is a prime ideal since the latter ring is a field.
We have thus shown that any non-nilpotent element is in the complement of
some prime ideal; the converse, that a nilpotent element belongs to all prime
ideals, is also clear. Hence the intersection of all prime ideals is also the ideal
consisting of all nilpotent elements of a ring; this ideal is called the nil radical of the
ring.
1.11. The Nilpotency of the Nil radical of a Noetherian Ring. When the
ring under consideration is Noetherian, the nil radical is finitely generated so
there is an upper bound k on the index of nilpotency of the generators. Let x1,
..., xl be the generators of the nil radical and consider an expression of the
form
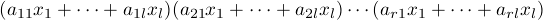
When r > l(k - 1) we see that each term in the expanded expression must contain the
k-th power of xi for at least one i. It follows that this expression must be zero whatever
the choice of the aij. This shows that the l(k - 1) + 1-th power of the nil radical
is the zero ideal. In other words the nil radical of a Noetherian ring is also
nilpotent.
1.12. When are Noetherian rings Artinian?. Let R be a Noetherian ring in which
there is just one prime ideal M; then that prime ideal is also a maximal ideal. As we
have seen above this prime ideal is the nil radical and is nilpotent; hence R = R/Ms for
some natural number s. If x1, ..., xr generate M then monomials of degree k
in the xi generate its k-th power Mk. This shows that Mk/Mk+1 is a finite
dimensional vector space over R/M for every k. It now follows that R is an Artinian
ring.
More generally, suppose R is a Noetherian ring in which every prime ideal
is a maximal ideal; let M be such an ideal. By what has been proved above
we see that the localisation RM of R with respect to the multiplicative set
R \ M is an Artinian ring; moreover we have RM = RM/MkRM = R/Mk for
some integer k. Let yM be an element of R whose image in this Artinian ring
is a minimal element; in other words AnnRM(yM) = MRM. It follows that
AnnR(yM) = M.
Consider the collection of elements yM of R as M varies over maximal ideals of R. By
the Noetherian-ness of R there is a finite collection yi = yMi;1 < i < p, such that every
yM is a linear combination of these yi; but then M = Ann(yM) contains the intersection
of Ann(yi) = Mi. It follows that M = Mi for some i. This proves that there are only
finitely many maximal (prime) ideals in R.
As seen above the nil radical of a Noetherian ring is nilpotent. Since every prime ideal
in our ring is maximal and there are only finitely many maximal ideals the nil radical is
of the form M1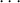 Mp. Thus there is an integer k such that M1k
Mp. Thus there is an integer k such that M1k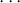 Mpk = 0. But then
we see that R = R/M1k ×
Mpk = 0. But then
we see that R = R/M1k ×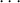 ×R/Mpk. Since each of the latter rings is Artinian we see
that R is Artinian.
×R/Mpk. Since each of the latter rings is Artinian we see
that R is Artinian.
1.13. Summary of Results. To summarize the results of this section:
Finite rings and finite dimensional rings are examples of
Artinian rings.
The Jacobson radical of an Artinian ring is the product of
its (finite collection of) maximal ideals and is a nilpotent ideal;
each prime ideal is maximal and consists of zero-divisors; the
complement of the union of all maximal ideals consists of units.
Essentially equivalently:
An Artinian ring A can be written in the form
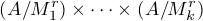
where the Mi are the (finite collection of) maximal ideals of A.
The groups Mip/Mip+1 are finite dimensional vector spaces over
the field A/Mi for each i and for each p.
Consequently:
An Artinian ring is also Noetherian.
Finally:
A Noetherian ring in which every prime is maximal is Artinian.
Appendix A. Some common applications of the structure of Artinian rings
The structure result for Artinian rings says that an Artinian ring has the form
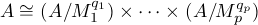
This result says that the idempotents of the ring A/M1 × × A/Mp can be lifted to
idempotents in the ring A. Most results on Artinian rings say that such liftings are
possible for various algebraic structures.
× A/Mp can be lifted to
idempotents in the ring A. Most results on Artinian rings say that such liftings are
possible for various algebraic structures.
A.1. Hensel’s Lemma. Let f(X) be a monic polynomial with coefficients in an
Artinian local ring A. Suppose that there are polynomials g(X) and h(X) in A[X] such
that f(X) - g(X)h(X) lies in M[X] where M is the Jacobson radical of M. Further
assume that g(X) and h(X) are co-prime modulo M; in other words we can find a(X)
and b(X) such that
![1 =_ a(X)g(X) + b(X)h(X) in (A/M )[X]](finite16x.png)
We also get a decomposition
![--
(A/M )[X]/(f(X)) = (A/M )[X]/(g(X))× (A/M )[X]/(h(X))](finite17x.png)
Since f(X) is a monic polynomial A[X]/(f(X)) is isomorphic to Adeg(f) as an
A-module; it follows that it is Artinian as an A-module and thus also as a ring. We will
now see how the idempotents corresponding to the above decomposition can be explicitly
lifted by factoring f(X).
Suppose we have found gk(X) and hk(X) in A[X] such that f(X) -gk(X)hk(X) lies
in Mk[X]. Moreover, assume that the images of gk(X) -g(X) and hk(X) -h(X) lie in
M[X]. Consider f(X) - gk(X)hk(X) as an element of (Mk/Mk+1)[X]; the latter is a
module over (A/M)[X]. Thus
Now
put We see that f(X) - gk+1(X)hk+1(X) lies in Mk+1[X]. Starting with k = 1 we see that
we can factor the image of f(X) in (A/Mk)[X] for all k.
Since A is Artinian local we see that A = A/Mk for some k. It follows that we have a
factoring of f(X) in A[X].
A.2. Weierstrass’ preparation theorem. Let f(X) be a formal power series over a
ring A. If the constant term of f is a unit in A, then by multiplying by its inverse we can
assume that the constant term of f is 1; thus f(X) = 1 -Xf0(X). If we write the formal
inverse
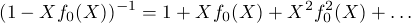
we can see that, for each positive integer k, the coefficient of Xk involves only finitely
many coefficients of f0(X). It can thus be computed as a formal power series g(X) in
A. To summarise, a formal power series with coefficients in A whose leading
coefficient is a unit in A, is also a unit in the ring of formal power series over
A.
Now, let K be a field and f(X) be any formal power series over K. Consider the first
integer n such that the coeffient of Xn is non-zero. Then f(X) = Xnf0(X) and by what
has been seen above f0(X) is a unit (since any non-zero element of a field is a unit).
Weierstrass’ preparation theorem generalises this to an appropriate statement for all
power series over an Artinian local ring.
Let f(X) be a formal power series with coeffients in an Artinian local ring A. Assume
that n is the first integer such that the coefficient of Xn in f(X) is a unit. For any power
series g let L(g) be the polynomial of degree at most n - 1 consisting of terms of
degree at most n - 1 of of g and H(g) = (g - L(g))/Xn be the high degree
terms of g. The given condition on f can be restated by saying that H(f) is a
unit in the ring of formal power series and L(f) is in M[X] where M is the
maximal ideal of the ring A. Let m = -L(f)H(f)-1; this is a formal power series
all of whose coefficients are in M; we have Xn = H(f)-1(X)f(X) + m(X).
Given any formal power series g(X) let us write M(g) = H(mg). We can write
We
have thus written g(X) = h1(X)f(X) + g1(X) + l1(X), where h1(X) is a power
series,g1(X) is a power series with coeffients in M and l1(X) is a polynomial of degree at
most (n- 1). Suppose that we have written g(X) = hk(X)f(X) + gk(X) + lk(X) where
hk(X) is a power series, gk(X) is a power series with coefficients in Mk and lk(X) is a
polynomial of degree at most (n - 1). We use the above formalism to write
So that putting hk+1 = hk + H(gk)H(f)-1, gk+1(X) = H(gk)(X)m(X) and
lk+1 = lk + L(gk), we see that we have the same formulae for g with k replaced by k + 1;
further note that gk+1(X) has coefficients in Mk+1 since the coefficients of H(gk) are the
same as the coefficients of gk and lie in Mk while the coefficients of m(X) are in M.
Since A is an Artinian ring A = A/Mk for some k; so gk(X) = 0. Thus we have obtained
an expression
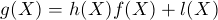
where h(X) is a power series and l(X) is a polynomial of degree at most n - 1.
Applying this to g(X) = Xn we see that Xn = u(X)f(X) + r(X). Comparing
coeffiecients of degree at most n- 1 on both sides we see that the coefficients of r(X) are
linear combinations of coefficients of degree at most n - 1 of f(X); thus r(X) lies in
M[X]. Further comparing the coeffiecients of Xn on both sides we see that the constant
coefficient of u(X) multiplied by the coefficient of Xn in f(X) differs from 1 by an
element of M. Thus the leading coefficient of u(X) is a unit and so u(X) is a
unit. We can also re-write the above equation as u(X)f(X) = Xn - r(X); in
words, any power series, with coefficients in an Artinian ring A, which is not
zero modulo M, is the multiple of a monic polynomial by an invertible power
series.
 I1
I1 
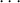 is constant after some i. A ring
satisfying this condition is also called an Artinian ring in honour of Emil Artin. The ring
also satisfies the ascending chain condition; any ascending chain of ideals is constant
after a finite stage. A ring satisfying this condition is called a Noetherian ring in honour
of Emmy Noether.
is constant after some i. A ring
satisfying this condition is also called an Artinian ring in honour of Emil Artin. The ring
also satisfies the ascending chain condition; any ascending chain of ideals is constant
after a finite stage. A ring satisfying this condition is called a Noetherian ring in honour
of Emmy Noether.
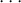 . By the descending chain condition this
sequence of ideals must become constant after some stage. In other words, there must be
an element
. By the descending chain condition this
sequence of ideals must become constant after some stage. In other words, there must be
an element 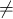 0 then we see that
0 then we see that 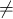 0; since (
0; since (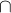


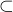
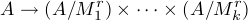
 , this sequence
is constant after some
, this sequence
is constant after some 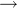
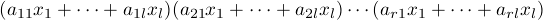
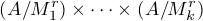
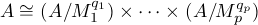
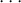
![1 =_ a(X)g(X) + b(X)h(X) in (A/M )[X]](finite16x.png)
![--
(A/M )[X]/(f(X)) = (A/M )[X]/(g(X))× (A/M )[X]/(h(X))](finite17x.png)